জটিল সংখ্যা
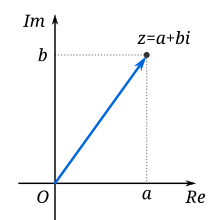
জটিল সংখ্যা (ইংৰাজী: Complex number) গণিতৰ কিছুমান সমস্যাৰ সমাধানৰ উদ্দেশ্যে গৃহীত এক ধাৰণা। সংখ্যাৰেখাৰ উলম্ব দিশত এক কাল্পনিক মাত্ৰাৰ (dimension) সহায়ত ইয়াক এটা বাস্তৱ সংখ্যা আৰু এটা কাল্পনিক সংখ্যাৰ ভেক্টৰ হিচাবে প্ৰকাশ কৰা হয়। [1]:
প্ৰতিটো জটিল সংখ্যাকেই আকাৰে লিখা যায়, য’ত a আৰু b বাস্তৱ সংখ্যা। a আৰু b-ক যথাক্ৰমে জটিল সংখ্যাৰ বাস্তৱ অংশ আৰু কাল্পনিক অংশ বোলা হয়।
জটিল সংখ্যাবোৰে এখন ফিল্ড তৈয়াৰ কৰে। এই কাৰণে ইয়াৰ ওপৰত যোগ, বিয়োগ, গুণ আৰু ভাগ, এই চাৰিটা দ্বিমিক অপাৰেশ্যন প্ৰয়োগ কৰা সম্ভৱ। এই জটিল সংখ্যাৰ অপাৰেশ্যনবোৰ বাস্তৱ সংখ্যাৰ অপাৰেশ্যনবোৰৰেই সম্প্ৰসাৰিত ৰূপ। কিছুমান জটিল সংখ্যাৰ ওপৰত প্ৰয়োগ কৰাৰ সময়ত এইবোৰ অপাৰেশ্যনৰ আৰু কিছু সুন্দৰ আৰু কাৰ্যকৰী বৈশিষ্ট্য পৰিলক্ষিত হয়। যেনে, কিছু জটিল (কাল্পনিক) সংখ্যাক বৰ্গ কৰি ঋণাত্মক বাস্তৱ সংখ্যা পোৱা যায়।
ইটালীয় গণিতিবিদ জিৰোলামো কাৰ্দানোয়ে ত্ৰিঘাত সমীকৰণ সমাধান কৰি থকা অৱস্থাত প্ৰথম জটিল সংখ্যা আবিষ্কাৰ কৰিছিল[2]। তেতিয়া ইয়াক "কাল্পনিক" উপাধি দিছিল।
জটিল সংখ্যাৰ যোগ, বিয়োগ, গুণ আৰু ভাগৰ নিয়ম প্ৰথমতে তৈয়াৰ কৰিছিল ইটালীয় গণিতবিদ ৰাফায়েল বোমবেল্লিয়ে। আইৰিশ গণিতবিদ উইলিয়াম ৰোয়ান হেমিল্টনে জটিল সংখ্যাৰ আৰু বিমূৰ্ত এটি বিধিবদ্ধ ৰূপ দিয়ে। তিনি জটিল সংখ্যাৰ তত্ত্বকে চতুষ্টিৰ তত্ত্বলৈ উন্নীত কৰে।
তড়িৎচৌম্বকত্ব, কোৱাণ্টাম পদাৰ্থবিজ্ঞান, ফলিত গণিত, বিশৃঙ্খলা তত্ত্ব ৰ বাহিৰেও প্ৰকৌশলৰ বিভিন্ন ক্ষেত্ৰত জটিল সংখ্যাৰ প্ৰচুৰ ব্যৱহাৰ হয়। কিছু কিছু ক্ষেত্ৰত নামৰ পৰাও জনা যায় যে সেইবোৰত অন্তৰ্নিহিত গাণিতিক সংগঠন হিচাপে জটিল সংখ্যাৰ ব্যৱহাৰ হৈছে। যেনে জটিল বিশ্লেষণ, জটিল মেট্ৰিস্ক, জটিল বহুপদী আৰু জটিল বীজগণিত।
তথ্যসূত্ৰ
[সম্পাদনা কৰক]- ↑ K. D. Joshi, Foundations of Discrete Mathematics, 1989, Wiley, p. 398 ISBN 0-470-21152-0
- ↑ Burton, David (1995). "7". The History of Mathematics (3rd সম্পাদনা). প্ৰকাশক New York: McGraw-Hill. পৃষ্ঠা. 294. ISBN 0-07-009465-9.
গাণিতিক তথ্যসূত্ৰ
[সম্পাদনা কৰক]- Ahlfors, Lars (1979), Complex analysis (3rd সম্পাদনা), McGraw-Hill, ISBN 978-0070006577
- Conway, John B. (1986), Functions of One Complex Variable I, Springer, ISBN 0-387-90328-3
- Pedoe, Dan (1988), Geometry: A comprehensive course, Dover, ISBN 0-486-65812-0
ঐতিহাসিক তথ্যসূত্ৰ
[সম্পাদনা কৰক]- Nahin, Paul J. (1998), An Imaginary Tale: The Story of (hardcover সম্পাদনা), Princeton University Press, ISBN 0-691-02795-1
- A gentle introduction to the history of complex numbers and the beginnings of complex analysis.
- H.-D. Ebbinghaus, H. Hermes, F. Hirzebruch, M. Koecher, K. Mainzer, J. Neukirch, A. Prestel, R. Remmert (1991), Numbers (hardcover সম্পাদনা), Springer, ISBN 0-387-97497-0
- An advanced perspective on the historical development of the concept of number.
বিতংকৈ পঢ়ক
[সম্পাদনা কৰক]- The Road to Reality: A Complete Guide to the Laws of the Universe, by Roger Penrose; Alfred A. Knopf, 2005; ISBN 0-679-45443-8. Chapters 4-7 in particular deal extensively (and enthusiastically) with complex numbers.
- Unknown Quantity: A Real and Imaginary History of Algebra, by John Derbyshire; Joseph Henry Press; ISBN 0-309-09657-X (hardcover 2006). A very readable history with emphasis on solving polynomial equations and the structures of modern algebra.
- Visual Complex Analysis, by Tristan Needham; Clarendon Press; ISBN 0-19-853447-7 (hardcover, 1997). History of complex numbers and complex analysis with compelling and useful visual interpretations.
পৰিভাষা
[সম্পাদনা কৰক]- Mathematical expansion - গাণিতিক সম্প্ৰসাৰণ
- Binary operation - বাইনাৰী প্ৰক্ৰিয়া
- Theory of Quarternion - চতুষ্টিৰ তত্ত্ব
- Associative rule - সহযোগী বিধি
- Commutative rule - বিনিময় বিধি
- Distributive rule - বণ্টন বিধি
- Algebraic structure - বীজগাণিতিক সংগঠন
- Additive identity - যোগাত্মক অভেদ
- Multiplicative identity - গুণাত্মক অভেদ
- Additive inverse - যোগাত্মক বিপৰীত
- Multiplicative inverse - গুণাত্মক বিপৰীত
- Field - ফিল্ড
- Subfield - উপফিল্ড
- Algerbraic number - বীজগাণিতিক সংখ্যা
- Topological closure - টপোগাণিতিক আৱদ্ধতা
- Algebraic closure - বীজগাণিতিক আৱদ্ধতা
বহিঃসংযোগ
[সম্পাদনা কৰক]- Euler's work on Complex Roots of Polynomials at Convergence
- John and Betty's Journey Through Complex Numbers
- Weisstein, Eric W., "Complex Number" মেথৱৰ্ল্ডৰ পৰা.
- "Argand Diagram" by Eric W. Weisstein, The Wolfram Demonstrations Project, 2007, and Argand Diagram on Weisstein's MathWorld.
- Complex Numbers Module by John H. Mathews
| |||||||||||













